Lesson Goal/Objectives: The objective for this lesson is for students to create a representation of a fractional part of a distance. Then, from a given assigned value of one fractional piece, determine the fractional distance remaining to reach the end goal and the total value of the whole distance using physical tools or a drawing. Students will show the thinking process used to establish the distance travelled and how they determined the total distance. Students will use physical tools of their choice to model their representation or draw a picture of their thinking. Students will then explain their thinking and representation using written words, and write the final answer in numerical form as a mixed number.
Original Task:
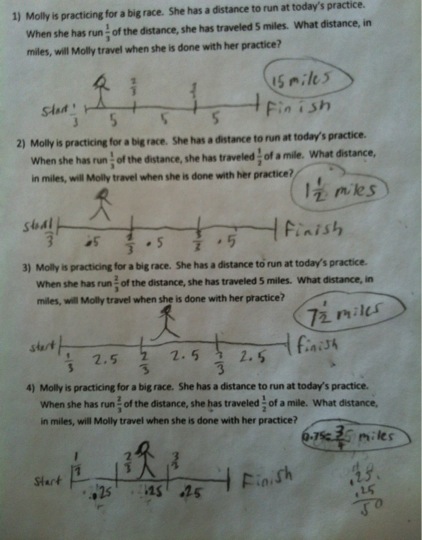
“Molly is practicing for a big race. She has a distance to run at today’s practice. When she has run 1/3 of the distance, she has travelled ½ mile. What distance, in miles, will Molly travel when she finishes her practice?”
Modified task:
“Molly is practicing for a big race. She has a distance to run at today’s practice. When she has run 1/3 of the distance, she has travelled 5 miles. What distance, in miles, will Molly travel when she finishes her practice?”
Essential Math Background Needed:
- Students need to have the ability to model a fraction.
- Students need to have an understanding of the part/whole relationship in order to manipulate values between fractional pieces.
- Students need to have a basic understanding of adding fractions within a whole with like denominators.

Virginia Mathematics Standards:
MTH.G3.3.a.1 – The student will name and write fractions (including mixed numbers) represented by a model to include halves, thirds, fourths, eighths, tenths, and twelfths.
MTH.G3.3.b.1 – Use concrete materials and pictures to model at least halves, thirds, fourths, eighths, tenths, and twelfths.
MTH.G3.7.a.1 – Demonstrate a fractional part of a whole, using: region/area models (e.g., pie pieces, patterns blocks, geoboards, drawings), set models (e.g., chips, counters, cubes, drawings), and length/measurement models (e.g., nonstandard units such as rods, connecting cubes, and drawings).
MTH.G3.7.a.3 – Represent a given fraction or mixed number, using concrete materials, pictures, and symbols. For example, write the symbol for one-fourth and represent it with concrete materials and/or pictures.
(Extending Indicator – Not essential knowledge expected to be taught by VDOE, but if students display grade level skills this indicator can be taught to extend grade level skills)
MTH.G3.7.a.5 – Create and solve word problems involving adding and subtracting fractions having like denominators
Students will begin at their seats for an individual warm-up activity modeling fractional parts to activate prior knowledge. Students will then gather on the front carpet for a whole group explanation of the day’s task and expectations while performing the task. Students will return to their seats for individual work, followed by a share time with partners, then time to go back and revise their own representations if they choose. The task will conclude with a group discussion of the day’s thinking process strategies.
Challenging Task presented in a 6th grade classroom
Foundational Mathematical Ideas: Fractions are defined as a part or piece of one whole unit. One whole can be divided into equal pieces to create equal fractional values. The representation of a fraction will change in value depending on what your baseline is for one whole unit. By combining fractional parts, you can create one whole unit and find the value for that whole by combining the value represented by each fractional piece. The advanced task requires students to split the value of two thirds into two equal values of one third each, and split the represented value of two thirds into two equal values as well. Students would then add the values together with the remaining value of the third to find the sum of all three thirds.
Real World Connections or Applications: When measuring length for spatial positioning within a region; constructing shelves, furniture, toys, units, etc.; reading maps for distance to figure out the best route of travel, allocating appropriate amounts of time when multiple tasks are required for completion within a given time period.
Vocabulary: Fraction, Region, Distance, Add, Value, Whole Unit, Representation, Model, Math Tools (Physical Tools)
Expected Prior Knowledge: Students will know how to divide one whole unit into equal fractional parts, represent a fraction with physical tools, assign equal values to equal fractional pieces, and add fractions with like denominators.
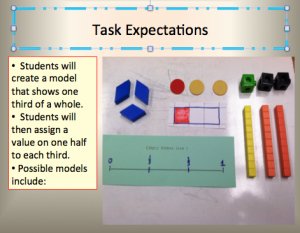
Launch/Warm-Up/Introduction: (12 Minutes) Students will be at their seats to begin the lesson. Students will be asked to represent one whole unit with fractional pieces and assign a value to each fractional piece. Students will be allowed to use any math tool they choose or draw a representation, and will have to explain to a partner how they represented one whole unit. Following the warm-up, students will gather on the front carpet for an introduction to the day’s task, and allotted time for each portion of the lesson. Expectations will be reviewed for usage of physical tools and individual work space.
Learning Activity: (21 Minutes) Students will return to their seats, and be given this task on a sheet of paper: “Molly is practicing for a big race. She has a distance to run at today’s practice. When she has run of the distance, she has travelled mile. What distance, in miles, will Molly travel when she finishes her practice?” The task paper will have a large empty work area, and dark lines across the bottom for students to explain their thinking using words. Math tools will be provided, including physical tools and graph paper. Students already have color pencils and/or crayons to use for drawings if they choose. This task was adapted from an original task created for higher grade level learners. Students will be given 21 minutes to begin solving the task. During this time, the teacher will navigate the room, prompting students with questions when assistance is requested, or deemed necessary. For students who solve the task prior to the 21 minute time allotment, they will be given a sheet of paper with a slight modification of the original task – written below. For students who have not found an entry point to the task given after 15 minutes, they will be given a sheet of paper with a modification of the task given at the beginning of the lesson – written below.
Group Discussion: (7 minutes) Students will be called to the carpet to discuss the task, particularly coming up with a variety of entry points for how students first approached the task. The purpose of the group discussion is to reinforce the idea of multiple entry points and the process skills behind students’ decision making. The answer to the task will not be discussed – so as to allow time for students who are still working to return to their seats and revise their representations.
Student Revisions: (10 minutes) During this time, students will be at their seats continuing to work on their task, and those students who have solved the task will be given the original, higher level task listed above. Students who have still not found an entry point will meet with the lead teacher to discuss possible strategies for finding an entry point – with the goal of being able to begin the process of setting up a possible representation to solve the task.
For students who solve the task prior to the 22 minute time allotment, they will be given the original task with only a whole number modification: “Molly is practicing for a big race. She has a distance to run at today’s practice. When she has run of the distance, she has travelled 8 miles. What distance, in miles, will Molly travel when she finishes her practice?” This modification to the original task challenges students to break the number eight into two equal parts. Students will be required to split the value of two thirds into two equal values of one third each, and split the represented value of eight miles into two equal values as well. Students would then add the values together with the value of the remaining third to find the sum of all three thirds. Prior knowledge needed includes addition strategies as well as using multiple representations within a task.
For students who have not found an entry point to the task given after 15 minutes, they will be given a sheet of paper with a modification of the task given: “Molly is practicing for a big race. She has a distance to run at today’s practice. When she has run of the distance, she has travelled 5 miles. What distance, in miles, will Molly travel when she finishes her practice?” This modification still requires students to use equal fractional parts to find a given distance, but scaffolds the distance travelled into whole numbers students are familiar using. In this case, the number 5, a number which students have already had background in adding and skip counting. If students have still not found an entry point to this task by the time we meet as a group to discuss strategies, those students will be pulled as a small group to discuss the modified task and develop possible entry points for the task.
Closure/Student Reflection:
(7 minutes) Students will regroup on the carpet to discuss the task. Focus points for discussion will include re-addressing the various entry points, and whether students were able to complete the task from those entry points. The discussion will then lead into what other types of real life tasks could this skill address – when you have a fractional part of a distance, and need to find how much is left.
(3 minutes) Students will then take a gallery walk to close the lesson for the opportunity to view the representations and written work of their classmates.
Informal/Observations – Throughout the lesson, the teacher will be monitoring student progress by navigating the room and looking for checkpoints of student progress: has the student found an entry point, has the student selected a representation strategy, has the student begun to create a physical/drawn representation, has the student begun to represent the task in words. The checklist will also include columns that allow for documentation if the student has selected an alternate entry point, and if the student chose to use a different representation strategy than the one originally chosen. Both of these columns will be split into two sub-columns: one sub-column to document a change made prior to the mid-point group discussion and one if the change was made during the revision period after the group discussion.
Handouts: For the teacher – Task Checklist for monitoring student progress during the task. For students – Modified Task to begin the lesson, Modified task for higher differentiation, Modified task with whole numbers for lower differentiation.
Additional Resources (if applicable): Physical math tools, including Base 10 blocks, pattern blocks, linking cubes, two color counters, graph paper, color pencils and crayons, and printed number lines without benchmarks.
EVALUATION POINTS DURING THE LESSON
Assessments/Rubrics – For the first five minutes of the student work period, the teacher will circulate the room to be certain that all students understand the language of the task, and understand what is being asked of them. The teacher will not discuss entry points, only explain the vocabulary of the task for clarity of purpose. After five minutes of work time, the teacher will then take the Rubric Checklist and monitor each student’s progress by checking if each student has found an entry point. The teacher will continue to monitor student progress for fifteen minutes, looking for signs of a physical or drawing tool selected, use of numbers, use of words, and completion of the task. After fifteen minutes, students who have not found an entry point will be given a similar task differentiated with whole numbers to facilitate accessibility. If those students with the whole number modification have still not found an entry point following the whole group entry point check-in, they will meet with the teacher in small group to discuss strategies for beginning the task.
All possible solution strategies:
Each third of any representations listed will have the value of: Fraction Task – ½, Whole Number Task – 5, Advanced Task – 4. Possible tools that can be used to represent the distance in thirds:
- Two Sided Counters (one chip red, two chips yellow – or vice versa)
- Linking Cubes (three cubes linked together, indicating thirds of a whole)
- Pattern Blocks (three blue rhombi in a row, if one whole is represented by a yellow hexagon)
- Graph Paper (any combination of squares split into three equal sizes)
- Number Line (a length divided into three equal distances)
- Base 10 Blocks (any three cubes, rods, or flats of the same size – indicating three equal parts of a distance)
- Student Drawings (any visual that shows three equal sections of one whole distance)
The student will show an addition sentence that indicates each third as an addend, and a cumulative sum of: Fraction Task – 1 ½ , Whole Number Task – 15, Advanced Task – 12.
Resources:
NSES Lesson Study Task ADVANCED
NSES Lesson Study Task Final Scoring Rubric
NSES Lesson Study Task FRACTIONS
NSES Lesson Study Task Teacher Checklist and Seating Chart
NSES Lesson Study Task WHOLE NUMBERS
NSES Lesson Study Warm Up Problem