Back to http://completecenter.gmu.edu/index.html

SOL Number and Number Sense
3.3 The student will a.) divide regions and sets to represent a fraction; and b.) name and write the fractions represented by a given model (area/region, length/measurement, and sent). Fractions (including mixed numbers) include halves, thirds, fourths, eighths, and tenths.
Goals:Students will be able to demonstrate an understanding of mixed numbers using words or pictures.
Students will use: problem-solving, mathematical communication, mathematical reasoning connections, mathematical representations
- Solidifying the concept of whole and equal parts, and introducing the concept of mixed numbers
- Students will have been exposed to the concept of a whole, and that fractions are used to name parts of a set.
Crestwood LessonStudy final Click here to view the instructional ppt
Key math vocabulary: whole, half, one-fourth or a quarter, equal, sharing, fractions
Refer back to previous sharing experience – Remembering Roberto’s birthday cupcakes. “Were there exactly the right amount? (There were leftovers) What if we wanted to eat all of the cupcakes, and make sure that everyone gets a fair share? Today we are going to introduce another Fair Share problem, but this time, we won’t have any leftovers, we are going to us fractions to show how the leftovers will be shared equally.”
Students at the carpet will be asked to divide one brownie between four people equally. Students will think-pair-share ideas to solve the problem.Teacher will select students who have the right idea based on what teacher hears.Teacher records accurate pictorial representation and numeric representation within a sentence frame “Each person gets ¼ of the whole brownie” for students to use as reference during the next task.ANTICIPATE: Possible Solutions for 4 people, 5 brownie sharing problem:
Possible solution 1: Explains as each person receiving 5/20 of a brownie.

Possible solution 2: Explains as each person receiving 5/4 of a brownie
Possible solution 3: Explains as each person receiving 1 ¼ of a brownie
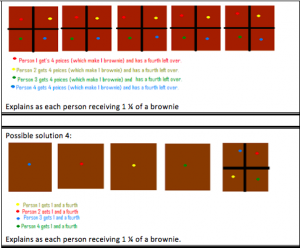
Possible solution 4: Explains as each person receiving 1 ¼ of a brownie.
Methods may include:.
- Dealing out the brownies like cards and dividing the last brownie into halves or 4ths.
- Using an algorithm.
- Saying that there is one left over.
- Drawing pictures.
Possible misconceptions:
Confusing the numerator with the denominator.
Once a brownie is divided, the parts are now wholes.
The last brownie cannot be divided.
Teacher asks:
How did you get 20? What does 20 represent?
Are those 20 pieces whole brownies? Or are they
fractions of brownies?
Resources:
1. Paper brownies
2. Scissors
3. Drawing paper
4. Markers
5. Chart paper
6. 5 sentence strips with pre-written sentence frame “Each person gets _______.”
Students will work individually and in pairs by ability and motivation. Students will record their work on the drawing paper.
Launch: After activating prior knowledge,Teacher will draw four faces and one rectangle on the white board to introduce four people sharing one brownie. Teacher will ask: How are they going to share that? What do I call each of these pieces? How can I write that as a fraction? How can I say that in words? Copy and finish the sentence on your own paper “Each person gets _______.” Teacher will record accurate pictorial and numeric responses.Now what if we have five brownies and four people? Think about how you can share them equally and represent that using fractions.Try to represent your thinking in words, pictures, and numbers.Teacher will introduce the challenge and thematerials available: newsprint, dark colored crayons, construction paper brownies, glue, scissors.Explore4 Kids, 5 Brownies (to share)
Students are each given the problem to solve individually, then share in their small groups.
– Teacher asks:
– What am I asking you to do?
– How can you represent your thinking? (Draw, write, write an equation)
– How did you find your answer? How can you represent that with numbers?
Show me how much each person gets.
What does this number represent?
What do we call this piece?
Can you write a fraction to show how much each person gets?
Can you write a sentence to show how much each person gets and explain your thinking?Follow-up discussion & record strategies used to solve the problem
– Kids share; selected by least to most efficient strategies.

+ Extensions:
(a) represent the problem using words and numbers (an equation)
(b) Teacher asks: How did Problem 1 help you to solve Problem 2? How are they the same? How are they different? Is there another way you can solve this? Can you come up with your own sharing problem? What happens if you have 5 Brownies and 3 people?
+ Refocusing:
Teacher asks: Show me how the brownies are being shared?
See some red questions above |
Lesson Closure (Selecting/ Sequencing):
Teacher questions to guide whole group discussion may include:
+ What does this number on the bottom represent?
+ What does this number on the top represent?
+ Are these two numbers the same?
+ Are these shared equally?
+ How can we represent that with words? With numbers?
+ Kids share; selected by least to most efficient strategies.
1. Least efficient is anticipated to be 5/202. Middle is anticipated to be dividing each into 4ths with a
total of 20 pieces , however they come to the conclusion that
it is 1 ¼.3. Most efficient: only dividing one brownie into 4ths and coming to 1 ¼.Students pair-share about their understanding of mixed numbers as a means of formative assessment after the whole group discussion.Teacher closes with: “Tomorrow we will discover even more about Mixed Numbers!” |
Connections:1. Least Efficient: Students understand that each person needs to have an equal share of each brownie2. Middle Group: Students understand that each part makes a whole; each ¼ makes 4/4.3. Most Efficient: Students understand that the relationship between fractions of a whole. Students understand that mixed numbers can be used to represent one whole and part of another whole.+ See: – Active engagement and discussion
– Representation shows an understanding of the problem and correct solution
+ Hear: – Students restating strategies used to solve the problem
– Students using the appropriate vocabulary when discussing those strategies
|
|
|
|
|
|
|
|
|




That insight solves the porbelm. Thanks!